
This article is meant to be read in a browser. There’s a PDF version made from Pandoc’s automatically generated latex, but this isn’t as nicely formatted as it would be if I’d typeset it by hand.
My mother wanted me to apply to Gonville & Caius College – which is usually just called “Caius” – because I had an uncle and grandfather who went there and she thought this might help me get in to Cambridge. These relatives had both studied engineering and this is what I applied to study too.
Whilst the practice of legacy admissions is still alive in the USA, I hope it wasn’t at Cambridge in 1966 when my application for admission was processed, but whatever the reason, I was very pleased to get an offer of a place and I was even awarded an entrance exhibition, which is a kind of minor scholarship.
I had very little idea of what engineering was like and during the nine months between being accepted and when I started as an undergraduate I came to realise that the subject was not what I wanted to spend the next three years immersed in, so I switched to mathematics. How I came to this view and managed to switch subjects is described in “How the gasworks changed my life”, which is the last section of the article on my gap year.
Students admitted to study mathematics are advised to do some preliminary reading as preparation for university level mathematics. One of the suggested books was Halmos’s ‘introduction’ to set theory.

Since I only switched subjects after arriving at Cambridge, I had done no preliminary reading. The school mathematics I’d learnt was very traditional – no hint of the New Math that was then popular – and although I knew a bit of formal logic and Venn diagram level set theory, I found the concept of axiomatic set theory, as covered in Halmos, unmotivated and almost impossible to understand.
It soon became clear that I was struggling to cope not only with the preliminary material I was assumed to know, but also with the content of the lectures, particularly pure mathematics such as analysis and abstract algebra. I was assigned extra supervisions by Peter Strain, a small and gentle graduate student with hair down to his waist; he would come to supervisions with a battered brown leather suitcase full of papers. Being curious about what happened to him I googled and it’s possible that he is this person and/or this one, but I can’t be sure.
Although I found the course very challenging, I also found it fascinating, even exciting, so I worked hard. I gradually got the hang of what modern mathematics is about and managed to get through the end of year exams – Part IA of the Mathematics Tripos – though with the lowest passing grade: Class III. My director of studies wrote a nice letter reassuring me that it was not a bad third, but the college felt that in view of my poor exam performance they would cancel my entrance exhibition.
For the long vacation after my first year I’d tried to get a summer job in a high tech company – what would now be called an internship – but got no offers. I signed on at the Camden Town labour exchange, but found nothing via them, and also found that I couldn’t get the dole (unemployment benefit). I ended up spending the summer living with my mother in Crouch End. This turned out to be a good thing because I had plenty of time for summer reading to catch up with the first year material and prepare for the second year of the course. Here are some of the books from then that I still have.

I particularly remember enjoying the book on measure theory by Kolmogorov & Fomin. After my summer of reading I returned to Cambridge able to survive the second year of the course.
The first two years of the Cambridge course required me to study both pure and applied mathematics, the latter consisting of advanced calculus and theoretical physics. During my first year I discovered that the pure topics had a much stronger allure than the applied ones. This is despite me having more background in applied mathematics because it was closer to stuff I’d studied at school. I found university pure mathematics new, exotic and mysterious, whilst the applied parts of the subject were just a much harder continuation of boring stuff I’d studied at school for my A-level exams. However, I may have survived my first year because I could at least understand what applied mathematics was. In my second year a lack of interest and ability meant that I struggled with applied the courses, particularly fluid dynamics.

Whilst I didn’t find the pure material easy, thanks to summer reading and my excitement with all the new ideas, I coped better with courses in these areas in my second year. I’d become a weak student rather than a failing one. I ended up with an improved grade in the second year exams.
My third and last year went well. I no longer had to study any theoretical physics and I could immerse myself in just a few courses of my own choosing.

I realised during my first two years that I was no good at mathematics that involved intricate reasoning or problem solving, but I had a taste for complex abstractions, as long as the ideas were fairly shallow. Most mathematics students like tricky problems and are good at solving then. Not me. I avoided topics like number theory that involved deductive intricacy. I only took courses that were built on layer upon layer of abstract definitions – e.g. manifolds, homology groups – and culminated in one big theorem like the classification of surfaces. Just mastering the standard concepts and theorems was enough work for an undergraduate course and exercises mostly depended only on understanding and regurgitating material from lecture notes. One couldn’t get far enough into the areas to enable novel tricky questions. This suited me as I enjoyed trying to grasp abstract concepts and following beautifully polished textbook proofs. I was pretty much incapable of original thinking, so found exercises involving this hard to impossible. I’ve memories of enjoyably struggling with courses on algebraic topology and differential geometry, both of which I recall as mostly consisting of towers of complicated definitions and constructions.
I obtained a better than expected result in the final exam, perhaps because I took courses that were examined with more bookwork questions than average … and I’d done the bookwork. I also did well in an experimental essay scheme which officially should not have influenced my exam result, but I suspect it did.
In the first year all lectures were given in the morning in Lecture Theatre A of the Arts School. Some lectures in later years were also given here, or in other rooms in the Arts School or elsewhere.

I have few memories of the teaching quality of particular courses. Mostly the lecturer copied pre-written notes onto the blackboard and we students copied these into a notebook. I found this worked well: during the lecture I got an overview of the main ideas and then later – usually in the afternoon after the lecture – I’d go through the notes and add explanatory annotations and formulate questions for upcoming supervisions.
One course I do remember was given by John Conway. I think it was on elementary analysis (\(\epsilon\)-\(\delta\) proofs, Rolle’s theorem, Mean Value Theorem etc.) and if so would have been in the first year. Conway didn’t copy pre-written notes onto the blackboard, but appeared to make up what he was saying as he went along. His style wasn’t smooth or rhetorically polished, but sometimes he seemed to spontaneously come up with quirky new ways of formulating definitions and proving theorems. He had a syllabus to cover, but he got through the material with a couple of lectures to spare, so he repeated the course just focusing on the main ideas and their connections. He got through this second version with half a lecture to spare, so he repeated the course for a third time by drawing a diagram on the blackboard consisting of all the key theorems with arrows between them representing entailments. I copied this graph structure down and memorised it. This was a fantastic tool for doing exercises of the form “prove \(A\Rightarrow B\)” because one could find the statements closest to \(A\) and \(B\) in the graph and then get an outline of the desired proof by finding a path between the statements.
The Cambridge Mathematical Tripos was too difficult for my abilities. I survived it, but I think I would have benefited from a gentler course.
I wouldn’t say that I was absolutely terrible at mathematics – after all I did manage to get a degree in it – but I’m definitely not a natural mathematician. Evidence for this is that I hated doing exercises: whenever possible I’d look up the answer rather than try to figure the solution out myself. Also, I have no interest in puzzles or recreational mathematics: I used to like perusing Scientific American, but rarely read Martin Gardner’s column.
It was fortunate that I came to the course with a realistic appreciation of my weaknesses. One of my first-year friends came from a large comprehensive school where he was the maths superstar. When he discovered that at Cambridge he was just average he got depressed and attempted unsuccessfully to commit suicide. He quit university and got a job. I only remember meeting him once after this, but he seemed happy. As mentioned before, and described in more detail elsewhere, I was aware from the beginning that by switching to mathematics I’d be in for a tough time. I never got depressed, though at the beginning I was scared that I’d fail my exams and be kicked out.
Each Cambridge undergraduate is required to become a member of a college. There are about 30 colleges and I became a member of Caius. The college supplies accommodation and meals. It also provides teaching support via supervisions; lectures are the responsibility of the university. Thus the people I lived, eat and studied with were from Caius, so formed my main social circle.
In the 1960s Caius was a traditional college. One had to wear an academic gown every day for dinner and when visiting ones Tutor, even for trivial administrative matters like getting an exeat. This sort of thing has fortunately largely disappeared now, but I found it pretty silly. I once called on my Tutor, John Casey, without a gown and got told off … however, when I grumbled, Casey explained the philosophico-ethical reasons why he thought such traditions should be preserved – this didn’t convince me, but I respected him for at least giving an analysis.
In the 1960s all Cambridge colleges were single sex; this, and the stuffy formal atmosphere of Caius, contrasted unfavourably with the trendy coeducational school I’d attended prior to university. I found it unnatural and insipid to live and work in a male-only environment. Even though I was a somewhat philistine science-oriented nerd at school, I missed its artsy intellectual atmosphere. Some of my schoolmates went to other colleges and these struck me as less stodgy and more fun than Caius. When I returned to Cambridge to study for the Diploma in Linguistics I switched to King’s College, which turned out not only to be more exciting and glamorous intellectually … and by then it also had women.
During my first and third year I lived in the still-to-be-refurbished Harvey Court, which was a modernist “seminal, Grade II* listed collegiate building”, but as a place to live was a gloomy brick fortress. The rooms were clean and comfortable, though the opposite of cosy.
My third year room had a huge south facing window that took up almost the whole wall and which I could walk through into a large and soothing garden where I’d pace around trying to understand mathematics. My first year room also had a walk through window, but this opened onto a barren brutalist plaza.
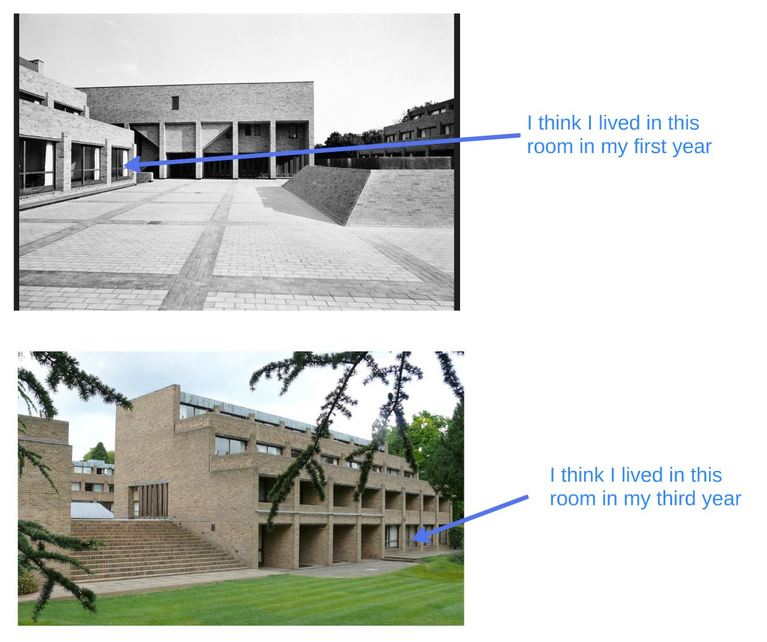
I gather that following a £7.5 million refurbishment in 2011 “for the demands of the twenty-first century student life” the gardens have shrunk, but from the pictures, it looks like the fortress gloom has been replaced by a light and airy ambience.
Not being a scholar, I couldn’t live in college for my second year. Together with some fellow Caius mathematics students, I found lodgings in Gresham Road. I’m pretty sure the address was 6 Gresham Road, which was – and still is – owned by the college, though it could have been an adjacent house. There was a landlady who lived on the ground floor and provided breakfast. I lived at the top of the house in a dingy but cosy two-room garret. It is here that necessity forced me to overcome my spider phobia.
Most afternoons I’d work in Gresham Road, breaking for tea and biscuits with the other people I knew in the lodgings. Each of us took turns to be host, make the tea and supply biscuits. Often we’d go to a local pub in the evenings. It is then that I learnt how to play bar billiards, though this isn’t a skill I’ve kept up with.
I’m pretty sure that switching from engineering to mathematics was a good move, but one can never be certain about such decisions. My three undergraduate years were not the best years of my life, but they weren’t awful. I made some good friends, but I didn’t stay in touch with them after I left Cambridge. Although I found the course very tough, it gave me the tools and confidence to feel that with sufficient effort – often more than I was willing to deploy – I could master any mathematical material I needed. This laid a solid foundation for my subsequent academic career. I’ve been more successful than I initially hoped and expected – there’s a good chance that the Mathematical Tripos should be thanked for this.
First complete draft: 10 September, 2017.