Linear Operators and Their Eigenfunctions

The above system h(t) is linear if it obeys the properties of superposition and proportionality:

• Superposition implies that if r₁(t) is the system's response to any input s₁(t), and if r₂(t) is the system's response to any input s₂(t), then the system's response to a third input s₃(t)=s₁(t)+s₂(t) which is the sum of the earlier two inputs, must simply be the sum of its responses to those two inputs separately: r₃(t)=r₁(t)+r₂(t).
• Proportionality implies that if any input s(t)is changed just by multiplying it by a constant k (which may be complex), then the system's original response r(t) simply gets multiplied by the same (possibly complex) constant: kr(t).

Linear systems are thus always described by some linear operator h(t). Examples of such linear operators are:

• Any derivative, or combination of derivatives of any order; any linear differential operator with constant coefficients.
• An integral expression.
• A convolution with some fixed waveform.
• Any combination or concatenation of the above.

The eigenfunctions of a system are those inputs which emerge completely unchanged at the output, except for multiplication by a constant (which may be complex). A fundamental property of linear systems as described above is that their eigenfunctions are the complex exponentials $\; \; \exp(i\mu_{k}t)$:

$\exp(i\mu_{k}t) \longrightarrow \; \;$ $\; \; \longrightarrow \; \; A\exp(i\mu_{k}t)$

That is, the only effect which a linear system h(t) can have on an input which is a complex exponential is to multiply it by a complex constant A when generating a response to it. Obviously, other families of input signals would become quite dramatically changed when operated upon by the sorts of linear operators ennumerated above. So, complex exponentials are a very special and important class of functions. 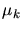In fact, if one can learn how a linear system h(t) responds to all possible complex exponentials (that is to say, if one can measure the complex constant A associated with every possible frequency $\mu_{k}$ of an input complex exponential), then one has complete knowledge about how the system will respond to any other possible input! This is an extraordinary kind of power.

The process works by representing any possible input as a superposition of complex exponentials, and then applying the superposition principles described earlier in order to calculate the output as another linear combination of those same complex exponentials, since they are eigenfunctions. In order to understand and apply this, we need to develop some of the tools of Fourier Analysis.